The following research was conducted by synthesizing high-quality, anonymous, first-person reports from psychedelic forums and reports sent directly to us. We do not condone the use of psychedelics because fully positive states cannot be guaranteed. For further reading on our views of psychoactive substances in the study of consciousness, see our research lineages. Content Warning: Trying to understand the contents of this essay may be mind-warping. Proceed with caution.
Friends, right here and now, one quantum away, there is raging a universe of active intelligence that is transhuman, hyperdimensional, and extremely alien. —Terence McKenna
The Geometry of DMT States
This is an essay on the phenomenology of DMT. The analysis here presented predominantly uses algorithmic, geometric and information-theoretic frameworks, which distinguishes it from purely phenomenological, symbolic, neuroscientific or spiritual accounts. We do not claim to know what ultimately(Emilsson 2015c) implements the effects here described (i.e. in light of the substrate problem of consciousness), but the analysis does not need to go there in order to have explanatory power. We posit that one can account for a wide array of (apparently diverse) phenomena present on DMT-induced states of consciousness by describing the overall changes in the geometry of one’s spatiotemporal representations (what we will call “world-sheets” i.e. 3D + time surfaces; 3D1T for short). The concrete hypothesis is that the network of subjective measurements of distances we experience on DMT (coming from the relationships between the phenomenal objects one experiences in that state) has an overall geometry that can accurately be described as hyperbolic (or hyperbolic-like). In other words, our inner 3D1T world grows larger than is possible to fit in an experiential field with 3D Euclidean phenomenal space (i.e. an experience of dimension R2.5 representing an R3 scene). This results in phenomenal spaces, surfaces, and objects acquiring a mean negative curvature. Of note is that even though DMT produces this effect in the most consistent and intense way, the effect is also present in states of consciousness induced by tryptamines(“Erowid Online Books : "TIHKAL" - the Continuation" by Alexander and Ann Shulgin,” n.d.) and to a lesser extent in those induced by all other psychedelics.
Conceptual Framework: Algorithmic Reduction
We will use the reduction framework originally proposed in the article Algorithmic Reduction of Psychedelic States(Emilsson 2016a). This means that we will be examining how algorithms and processes (as experienced by a subject of experience) can explain the dynamics of people’s phenomenology in DMT states. We do not claim “the substrate of consciousness” is becoming hyperbolic in any literal sense (though we do not discard that possibility). Rather, we interpret the hyperbolic curvature that experience acquires while on DMT as an emergent effect of a series of more general mechanism of action that can work together to change the geometry of a mind. These same mechanisms of action govern the dynamics of other psychedelic experiences; it is the proportion and intensity of the various “basic” effects that lead to the different outcomes observed. In other words, the hyperbolization of phenomenal space may not be a fundamental effect of DMT, but rather, it may be an emergent effect of more simple effects combined (not unlike how our seemingly smooth macroscopic space-time emerges from the jittery yet fundamental interactions that happen in a microscopic high-dimensional quantum foam).
In particular, we will discuss three candidate models for a more fundamental algorithmic reduction: (1) the synergistic effect of control interruption(“The Control Interrupt Model of Psychedelic Action,” n.d.) and symmetry detection resulting in a change of the metric of phenomenal space (analogously to how one can measure the geometry of hyperbolic graph embeddings), (2) the mind as a dynamic system with energy sources, sinks and invariants, in which curvature stores potential energy, and (3) a change in the underlying curvature of the micro-structure of consciousness. These models are not mutually-exclusive, and they may turn out to be compatible. More on this later.
What is Hyperbolic Geometry?
Perhaps the clearest way to describe hyperbolic space is to show examples of it:
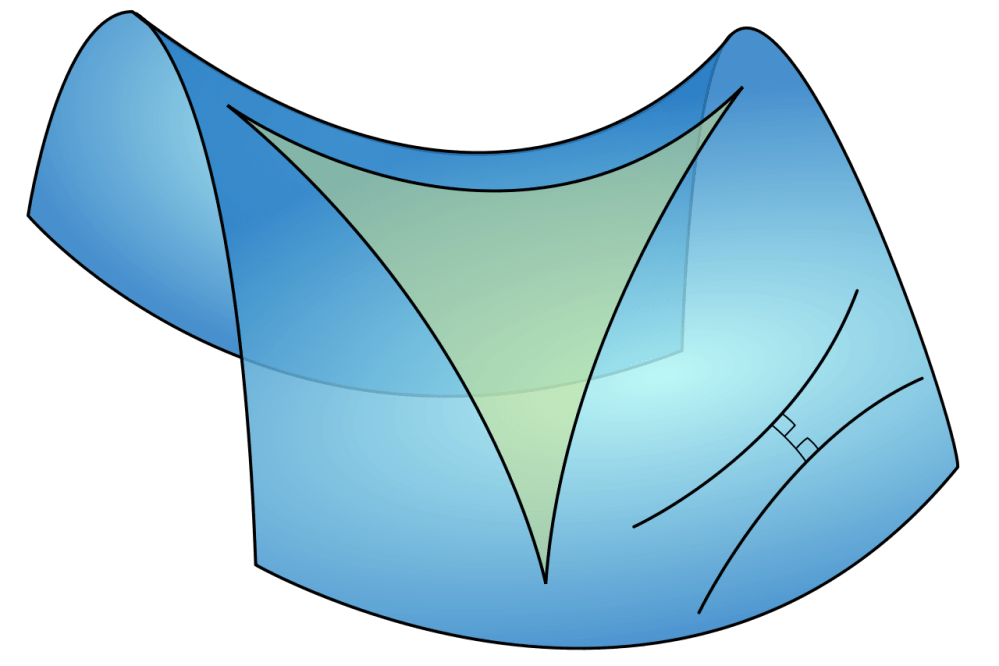
The picture to the left shows a representation of a “saddle” surface. In geometry, saddle surfaces are 2-dimensional hyperbolic spaces (also called “hyperbolic planes” or H2(“Visualizing the Sphere and the Hyperbolic Plane: Five Projections of Each,” n.d.)). For a surface to have “constant curvature” it must look the same at every point. In other words, for a saddle to be a geometric saddle, every point in it must be a “saddle point(“Saddle Points,” n.d.)” (i.e. a point with negative curvature(“Negative Curvature, Surface of - Encyclopedia of Mathematics,” n.d.)). As you can see, saddles have the property that the angles of a triangle found in them add up to less than 180 degrees (compare that to surfaces with positive curvature such as the 2-sphere, in which the angles of a triangle add up to more than 180 degrees). Generalizing this to higher dimensions, the middle image above shows a cube in H3 (i.e. a hyperbolic space of three dimensions). This cube, since it is in hyperbolic space, has thin edges and pointy corners. More generally, the corners of a polyhedra (and polytopes) will be more pointy in Hn than they are in Rn. This is why you can see in the right image a dodecahedron with right-angled corners, which in this case can tile H3 (cf. Not Knot(“Not Knot,” n.d.)). Such a thingpeople of the past might say- is an insult to the imagination. Times are changing, though, and hyperbolic geometry is now an acceptable subject of conversation.
An important property of hyperbolic spaces is the way in which the area of a circle (or the n-dimensional volume of a hypersphere) increases as a function of its radius. In 2D Euclidean space the area grows quadratically with the radius. But on H2, the area grows exponentially as a function of the radius! As you may imagine, it is easy to get lost in(“Hyperbolic Geometry in HyperRogue,” n.d.) hyperbolic space. A few steps take you to an entirely different scene. More so, your influence over the environment is greatly diminished as a function of distance. For example, the habitable region of solar systems in hyperbolic spaces (i.e. the Goldilocks zone) is extremely thin. In order to avoid getting burned or freezing to death you would have to place your planet within a very narrow distance range from the center star. Most of what you do in hyperbolic space either stays as local news or is quickly dissipated in an ever-expanding environment.
We Can Only Remember What We Can Reconstruct
We cannot experience H2 or H3 manifolds under normal circumstances, but we can at least represent some aspects of them through partial embeddings(Weisstein, n.d.) (i.e. instantiations as subsets of other spaces preserving properties) and projections(“Hyperbolic Space,” n.d.) into more familiar geometries. It is important to note that such representations will necessarily be flawed. As it turns out, it is notoriously hard to truly embed H2 in Euclidean 3D space, since doing so will necessarily distort some properties(“Visualizing the Sphere and the Hyperbolic Plane: Five Projections of Each,” n.d.) of the original H2 space (such as distance, angle, area, local curvature, etc.). As we will discuss further below, this difficulty turns out to be crucial for understanding why DMT experiences are so hard to remember. In order to remember the experience you need to create a faithful and memorable 3D Euclidean embedding of it. Thus, if one happens to experience a hyperbolic object and wants to remember as much of it as possible, one will have to think strategically about how to fold, crunch and deform such object so that it can be fit in compact Euclidean representations.
What about DMT Suggests Hyperbolic Geometry?
Why should we believe that phenomenal space on DMT (and to a lesser extent on other psychedelics) becomes hyperbolic-like? We will argue that the features people use to describe their trips as well as concrete mathematical observations of such features point directly to hyperbolic geometry. Here is a list of such features (arranged from least to most suggestive… you know, for dramatic effect):
- Perception of far-out travel (as we said, small movements in hyperbolic space lead to huge changes in the scene).
- Feelings of becoming big (you can fit a lot more inside a circle of radius r in hyperbolic space).
- The space experienced is often depicted as “more real and more dense than normal.”
- The use of terms like “mind-expanding” and “warping” to describe the effects of the drug are very common.
- People describing it as “a different kind of space” and frequently using the word “hyperspace” to talk about it.
- Difficulty integrating/remembering the objects and scenes experienced (e.g. “they were too alien to recall”).
- Constant movement/acceleration and change of perspectives which are often described as “unfolding scenes and expanding patterns” (cf. the chrysanthemum(“Chrysanthemum - First Steps in Hyperspace - Welcome to the DMT-Nexus,” n.d.), jitterbox(“The Jitterbox: 3d Auxetic Material,” n.d.)).
- Continuous change of the scene’s context through escape routes: A door that leads to a labyrinth that leads to branching underground tunnels that lead to mirror rooms that lead to endless windows, and the one you take leading you to a temple with thirty seven gates which lead you to a kale salad world etc. (example(“Experience:30mg Smoked DMT - the Monolith,” n.d.))
- Crowding of scene beyond the limits of Euclidean space (users frequently wondering “How was I able to fit so much in my mind? I don’t see any space for my experience to fit in here!”)
- Reported similarity with fractals.
- Omnipresence of saddles making up the structural constraints of the hallucinated scenes. For example, one often hears about experiencing scenes saturated with: joints, twists, bifurcations, curved alleys, knots, and double helixes.
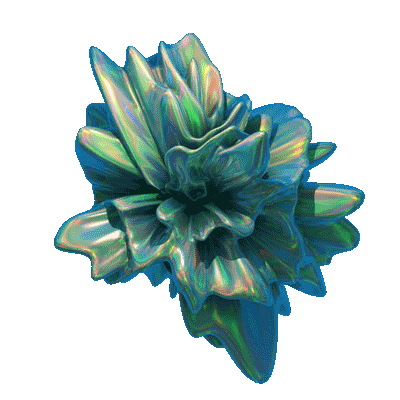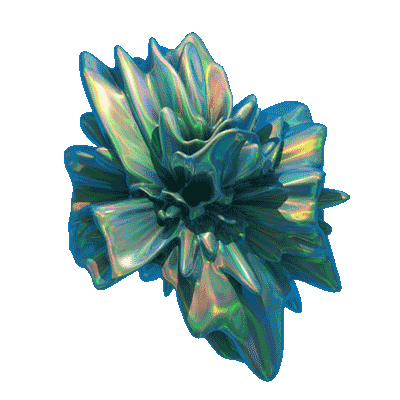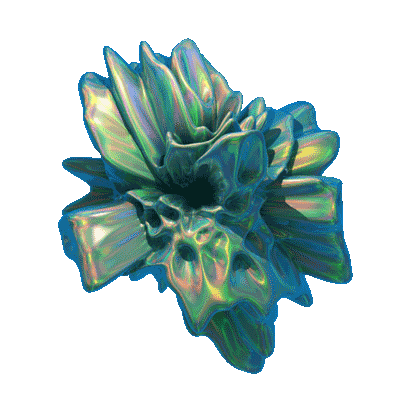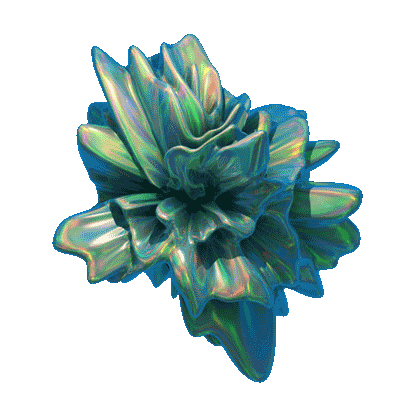
- Looking at self-similar objects (such as cauliflowers) can get you lost in what seems like endless space. (Note: beware of the potential side effects of looking at a cauliflower on DMT1).
- PSIS-like experiences(Emilsson 2016c) where people seem to experience multiple alternative outcomes from each event at the same time (this may be the result of “hyperbolic branching” through time rather than space).
- Psychedelic replication pictures usually include features that can be interpreted as hyperbolic objects embedded in Euclidean 3D(“Embedding a Torus (John Nash) - Numberphile,” n.d.).
- People describe “incredibly advanced mechanisms” and “impossible objects” that cannot be represented in our usual reality (e.g. Terence McKenna’s self-dribbling basketballs).
- At least one mathematician has stated that what one experiences on DMT cannot be translated into Euclidian geometry (unlike what one experiences on LSD).
- We received a series of systematic DMT trip-reports by a math enthusiast and experienced psychonaut who claims that the surfaces experienced on DMT are typically composed of hyperbolic tilings (which imply a negative curvature; cf. wallpaper groups).
This article goes beyond claiming a mere connection between DMT and hyperbolic geometry. We will be more specific by addressing the aspects of the experience that can be interpreted geometrically. To do so, let us now turn to a phenomenological description of the way DMT experiences usually unfold:
The Phenomenology of DMT Experiences: The Six Levels
In order to proceed we will give an account of a typical vaporized DMT experience. You can think of the following six sections as stages or levels of a DMT journey. Let me explain. The highest level you get to depends on the dose consumed, and in high doses one experiences all of the levels, one at a time, and in quick succession (i.e. on high doses these levels are perceived as the stages of the experience). If one takes just enough DMT to cross over to the highest level one reaches during the journey for only a brief moment, then that level will probably be described as “the peak of the experience.” If, on the other hand, one takes a dose that squarely falls within the milligram range for producing a given level, it will be felt as more of a “plateau.” Each level is sufficiently distinct from the others that people will rarely miss the transitions between them.
The six levels of a DMT experience are: Threshold, Chrysanthemum, Magic Eye, Waiting Room, Breakthrough, and Amnesia. Let us dive in!
(Note: The following description assumes that the self-experimenter is in good physical and mental health at the time of consuming the DMT. It is well known that negative states of consciousness can lead to incomprehensible hellscapes when “boosted” by DMT (please avoid DMT at all costs while you are drunk, depressed, angry, suicidal, irritable, etc.). The full geometry is best appreciated on a mentally and emotionally balanced set and settings.)
Threshold
The very first alert of something unusual happening may take between 3 to 30 seconds after inhaling the DMT, depending on the dose consumed. Rather than a clear sensorial or cognitive change, the very first hint is a change in the apparent ambiance of one’s setting. You know how at times when you enter a temple, an art museum, a crowd of people, or even just a well decorated restaurant you can abstract an undefinable yet clearly present “vibe of the place?” There’s nothing overt or specific about it. The ambiance of a place is more of an overall gestalt than a localized feeling. An ambiance somehow encodes information about the social, ideological and aesthetic quality of the place or community you just crashed into, and it tells you at a glance which moods are socially acceptable and which ones are discouraged. The specific DMT vibe you feel on a given session can be one of a million different flavors. That said, whether you feel like you entered a circus or joined a religious ceremony, the very first hint of a DMT experience is nonetheless always (or almost always) accompanied with an overall feeling of significance. The feeling that something important is about to happen or is happening is made manifest by the vibe of the state. This vibe is usually present for at least the first 150 seconds or so of the journey. Interestingly, the change in ambiance is shorter-lived than the trip itself; it seems to go away before the visuals vanish quickly declining once the the peak is over.
Within seconds after the change in ambiance, one feels a sudden sharpening of all the senses. Some people describe this as “upgrading one’s experience to an HD version of it.” The level of detail in one’s experience is increased, yet the overall semantic content is still fairly intact. People say things like: “Reality around me seems more crisp” and “it’s like I’m really grasping my surroundings, you know? fully in tune with the smallest textures of the things around me.” Terence McKenna described this state as follows: “The air appears to suddenly have been sucked out of the room because all the colors brighten visibly, as though some intervening medium has been removed.”

On a schedule of repeated small doses (below 4 mg; preferably i.m.) one can stabilize this sharpening of the senses for arbitrarily long periods of time. I am a firm believer that this state (quite apart from the alien experiences on higher doses) can already be recruited for a variety of computational and aesthetic tasks that humans do in this day and age. In particular, the state itself seems to enable grasping complex ideas with many parameters without distorting them, which may be useful for learning mathematics at an accelerated pace. Likewise, the sate increases one’s awareness of one’s surroundings (possibly at the expense of consuming many calories). I find it hard to imagine that artists will not be able to use this state for anything valuable.
The Chrysanthemum
If one ups the dose a little bit and lands somewhere in the range between 4 to 8 mg, one is likely to experience what Terrence McKenna called “the Chrysanthemum.” This usually manifests as a surface saturated with a sort of textured fabric composed of intricate symmetrical relationships, bright colors, shifting edges and shimmering pulsing superposition patterns of harmonic linear waves of many different frequencies.
Depending on the dose consumed one may experience either one or several semi-parallel channels. Whereas a threshold dose usually presents you with a single strong vibe (or ambiance), the Chrysanthemum level often has several competing vibes each bidding for your attention. Here are some examples of what the visual component of this state of consciousness may look like:
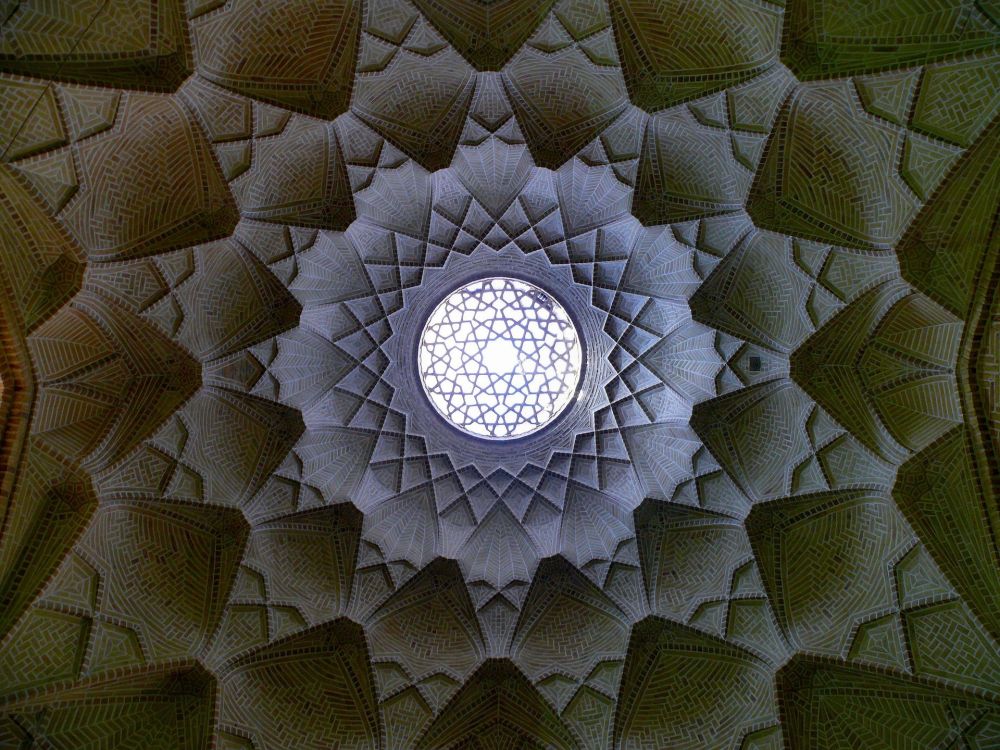


The visual component of the Chrysanthemum is often described as “the best screen saver ever(“Neuroscientist Describes Her DMT Trip,” n.d.),” and if you happen to experience it in a good mood you will almost certainly agree with that description, as it is usually extremely harmonious, symmetric and beautiful in uncountable ways. No external input can possibly replicate the information density and intricate symmetry of this state; such state has to be endogenously generated as a a sort of harmonic attractor of your brain dynamics.
You can find many replications of Chrysanthemum-level DMT experiences on the internet, and I encourage you to examine their implicit symmetries (this replication(“Descent into Madness,” n.d.) is one of my all-times favorite).
In Algorithmic Reduction of Psychedelic States(Emilsson 2016a) we posited that any one of the 17 wallpaper symmetry groups can be instantiated as the symmetries that govern psychedelic visuals. Unfortunately, unlike the generally slow evolution of usual psychedelic visuals, DMT’s vibrational frequency forces such visuals to evolve at a speed that makes it difficult for most people to spot the implicit symmetry elements that give rise to the overall mathematical structure underneath one’s experience. For this reason it has been difficult to verify that all 17 wallpaper groups are possible in DMT states. Fortunately we were recently able to confirm that this is in fact the case thanks to someone who trained himself to do just this. I.e. detecting symmetry elements in patterns at an outstanding speed.
An anonymous psychonaut (whom we will call researcher A) sent a series of trip report to Qualia Computing detailing the mathematical properties of psychedelic visuals under various substances and dose regimens. A is an experienced psychonaut and a math enthusiast who recently trained himself to recognize (and name) the mathematical properties of symmetrical patterns (such as in works of art or biological organisms). In particular, he has become fluent at naming the symmetries exhibited by psychedelic visuals. In the context of 2D visuals on surfaces, A confirms that the symmetrical textures that arise in psychedelic states can exhibit any one of the 17 wallpaper symmetry groups. Likewise, he has been able to confirm that every possible spherical symmetry group can also be instantiated in one’s mind on these states.
The images below show some examples of the visuals that A has experienced on 2C-B, LSD, 4-HO-MET and DMT (sources: top left(“Symmetry Stanford Vision and Neuro-Development Lab,” n.d.), top middle; the rest were made with this service(“PhiloGL - Wallpaper Groups,” n.d.)):
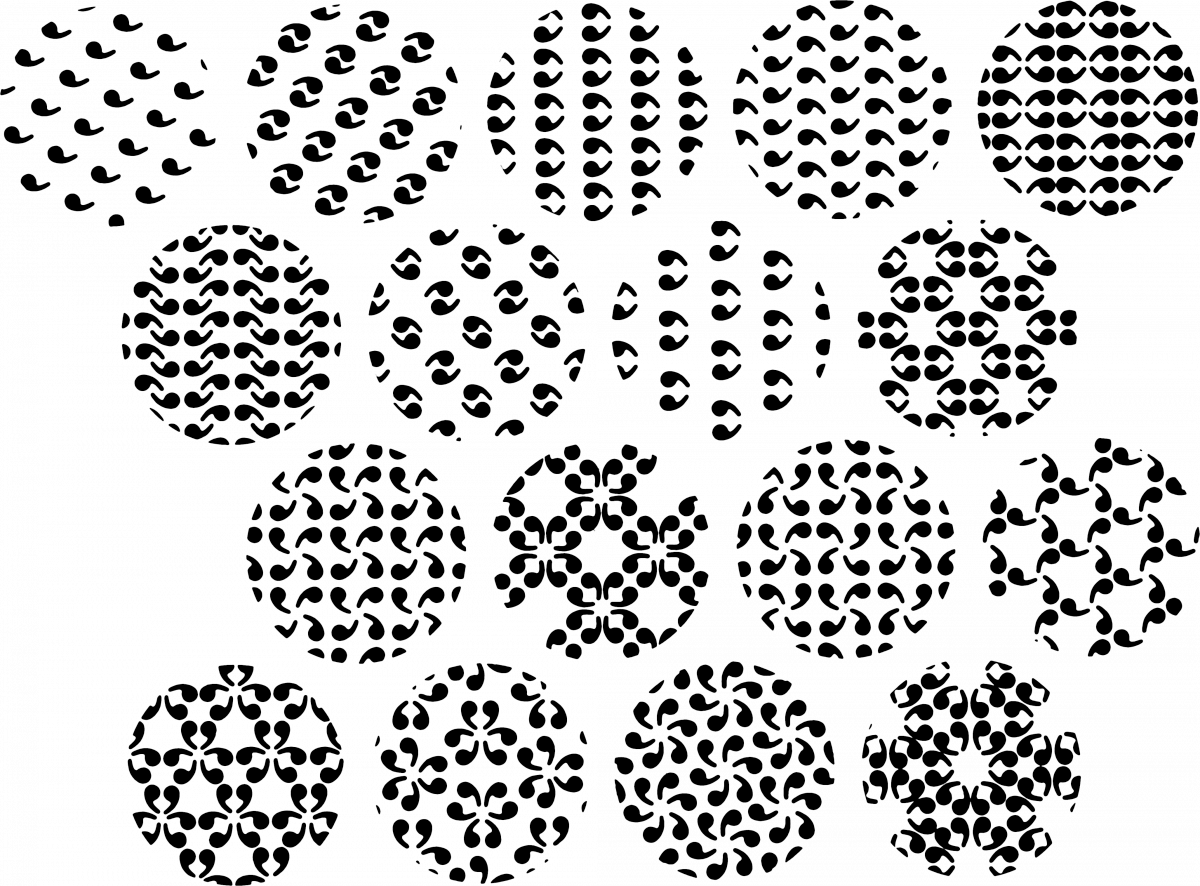



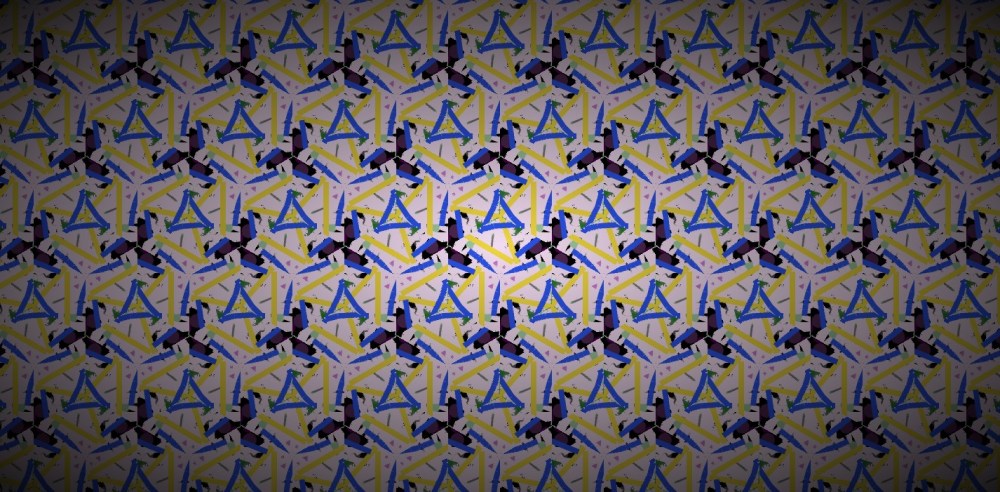

The Chrysanthemum level interacts with sensory input in an interesting way: the texture of anything one looks at quickly becomes saturated with nested 2-dimensional symmetry groups. If you took enough DMT to take you to this level and you keep your eyes open and look at a patterned surface(Emilsson 2015a) (i.e. statistical texture(Emilsson 2015b)), it will symmetrify beyond recognition. A explains that at this level DMT visuals share some qualities with those of, say, LSD, mescaline, and psilocin. Like other psychedelics, DMT’s Chrysanthemum level can instantiate any 2-dimensional symmetry, yet there are important differences from other psychedelics at this dose range. These include the consistent change in ambiance (already present in threshold doses), the complexity and consistency of the symmetrical relationships (much more dense and whole-experience-consistent than is usually possible with other psychedelics), and the speed (with a control interruption frequency(“The Control Interrupt Model of Psychedelic Action,” n.d.) reaching up to 30 hertz, compared to 10-20 hertz for most psychedelics). Thus, people tend to point out that DMT visuals (at this level) are “faster, smaller, more detailed and more globally consistent” than on comparable levels of alteration from similar agents.
Now, if you take a dose that is a little higher (in the ballpark of 8 to 12 mg), the Chrysanthemum will start doing something new and interesting…
The Magic Eye Level
A great way to understand the Magic Eye level of DMT effects is to think of the Chrysanthemum as the texture of an autostereogram (colloquially described as “Magic Eye” pictures). Our visual experience can be easily decomposed into two points-of-view (corresponding to the feed coming from each eye) that share information in order to solve the depth-map problem in vision. This is to map each visual qualia to a space with relative distances so (a) the input is explained and (b) you get recognizable every-day objects represented as implicit shapes beneath the depth-map. You can think of this process as a sort of handshake(Alex and er 2016) between bottom-up perception and top-down modeling.
In everyday conditions one solves the depth-map problem within a second of opening one’s eyes (minus minor details that are added as one looks around). But on DMT, the “low-level perceptions” looks like a breathing Chrysanthemum, which means that the top-down modeling has that “constantly shifting” stuff to play with. What to make of it? Anything you can think of.
There are three major components of variance on the DMT Magic Eye level: 1. Texture (dependent on the Chrysanthemum’s evolution) 2. World-sheet (non-occluding 3D1T depth maps) 3. Extremely lowered information copying threshold.



The image on the left is a lobster, the one on the center is a cone and the one to the right contains furniture (a lamp, a chair and a table). Notice that what you see is a sort of depth-map which encodes shapes. We will call this depth-map together with the appearance of movement and acceleration represented in it, a world-sheet.
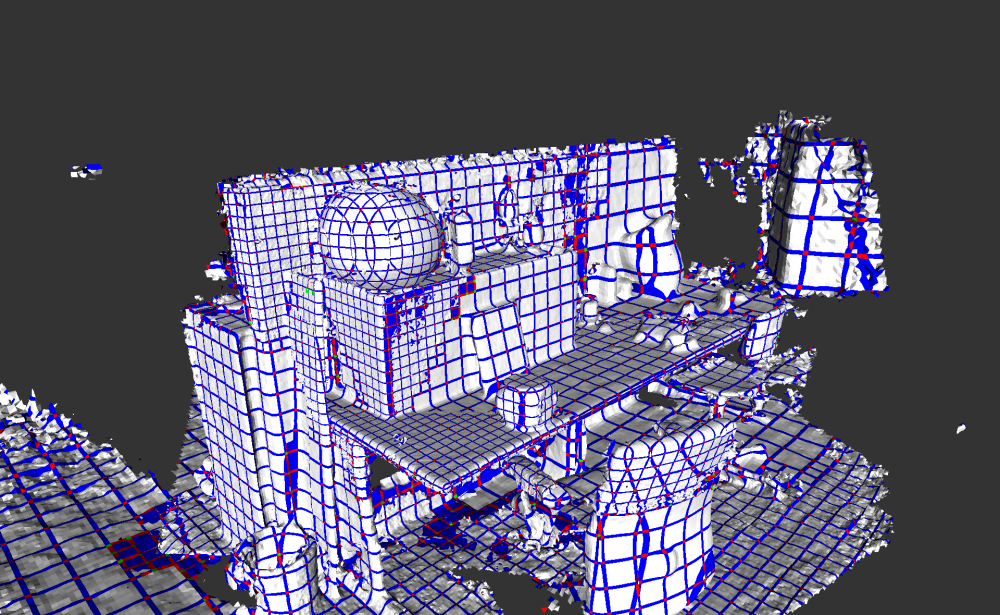

The world-sheet encodes the “semantic content” of the scene and is capable of representing arbitrary situations (including information about what you are seeing, where you are, what the entities there are doing, what is happening, etc.).
It is common to experience scenes from usually mundane-looking places like ice-cream stores, play pens, household situations, furniture rooms, apparel, etc.. Likewise, one frequently sees entities in these places, but they rarely seem to mind you because their world is fairly self-contained. As if seeing through a window. People often report that the worlds they saw on a DMT trip were all “made of the same thing.” This can be interpreted as the texture becoming the surfaces of the world-sheet, so that the surfaces of the tables, chairs, ice-cream cones, the bodies of the people, and so on are all patterned with the same texture (just as in actual autostereograms). This texture is indeed the Chrysanthemum completely contorted to accommodate all the curvature of the scene.
Magic Eye level scenes often include 3D geometrical shapes like spheres, cones, cylinders, cubes, etc. The complexity of the scene is roughly dose-dependent. As one ups the highness (but still remaining within the Magic Eye level) complex translucid qualia crystals in three dimensions start to become a possibility.
Whatever phenomenal objects you experience on this level that lives for more than a millisecond needs to have effective strategies for surviving in an ecosystem of other objects adapted to that level. Given the extremely lowered information copying threshold, whatever is good at making copies of itself will begin to tessellate, mutate and evolve, stealing as much of your attention as possible in the way. Cyclic transitions occupy one’s attention: objects quickly become scenes which quickly become gestalts from which a new texture evolves in which new objects are detected and so on ad infinitum.
A reports that at this dose range one can experience at least some of the 230 space groups as objects represented in the world-sheet. For example, A reports having stabilized a structure with a Pm-3m symmetry structure, not unlike the structure of ZIF-71-RHO. Visualizing such complex 3D symmetries, however, does seem to require previous training and high levels of mental concentration (i.e. in order to ensure that all the symmetry elements are indeed what they are supposed to be).
There is so much qualia laying around, though, at times not even your normal space can contain it all. Any regular or semi regular symmetrical structure you construct by focusing on it is prone to overflow if you focus too much on it. What does this mean? If you focus too much on, for example, the number 6, your mind might represent the various ways in which you can arrange six balls in a perfectly symmetrical way. Worlds made of hexagons and octahedrons interlocked in complex but symmetrical ways may begin to tesselate your experiential field. With every second you find more and more ways of representing the number six in interesting, satisfying, metaphorically-sound synesthetic ways (cf. Thinking In Numbers(Emilsson 2016e)). Now, what happens if you try to represent the number seven in a symmetric way on the plane? Well, the problem is that you will have too many heptagons to fit in Euclidean space (cf. Too Many Triangles(“Too Many Triangles - Numberphile,” n.d.)). Thus the resulting symmetrical patterns will seem to overflow the plane (which is often felt as a folding and fluid re-arrangement, and when there is no space left in a region it either expands space or it is felt as some sort of synesthetic tension or stress, like a sense of crackling under a lot of pressure).

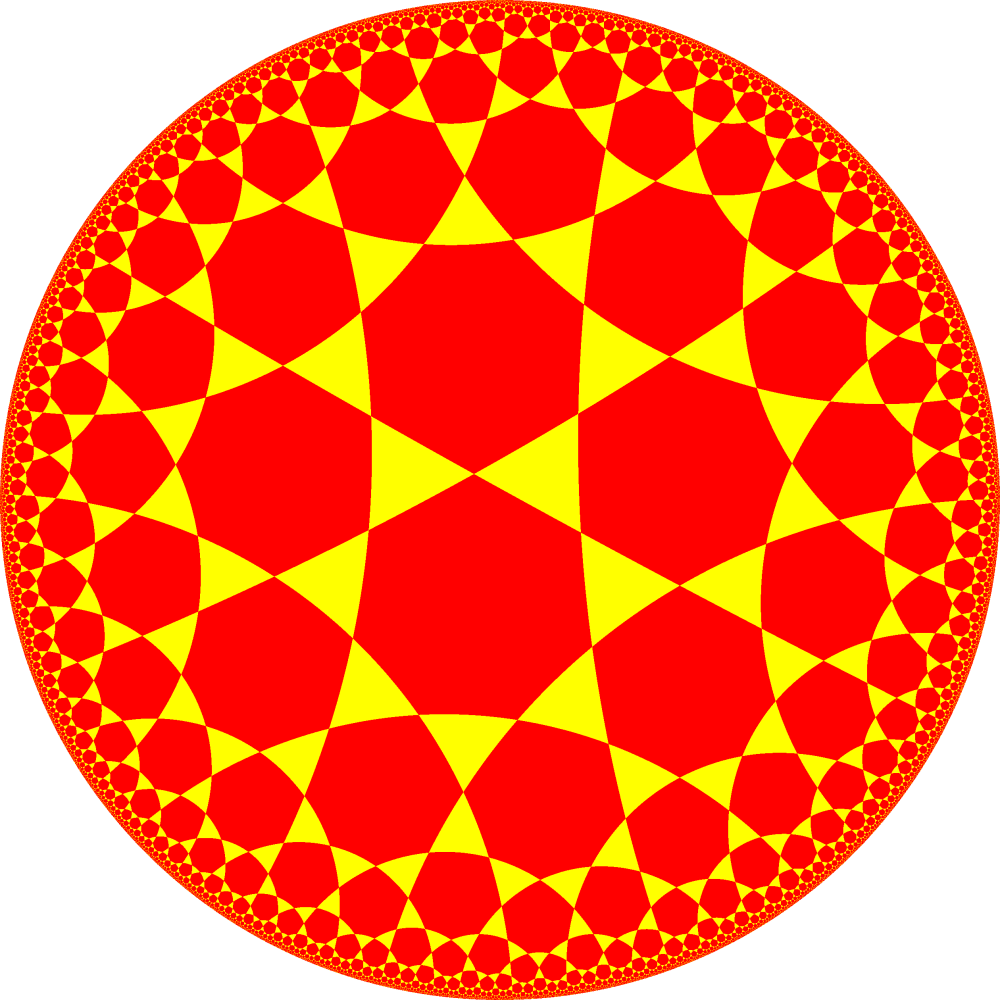
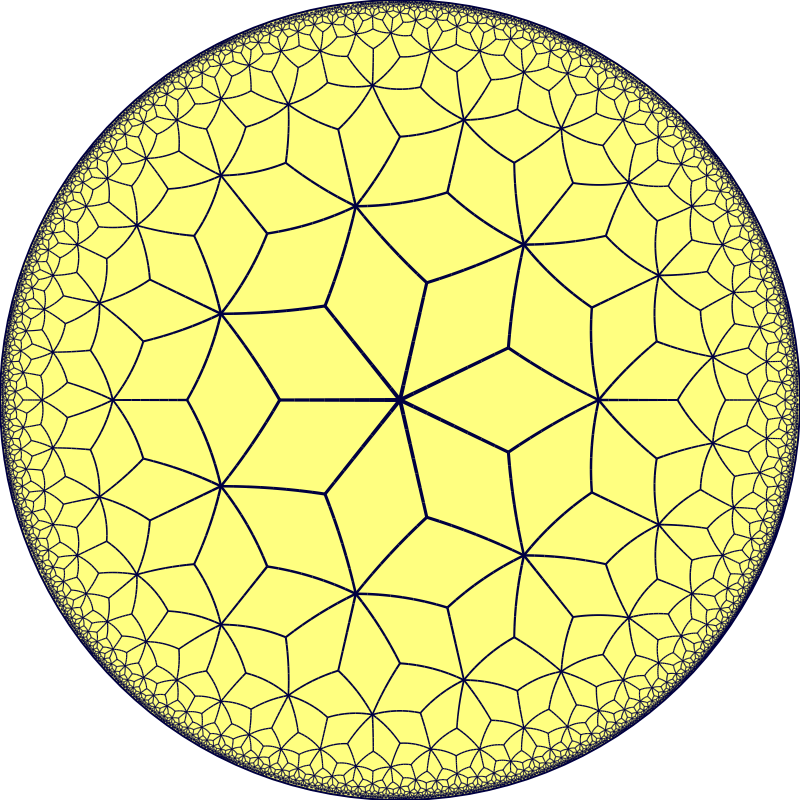
In particular, A claims that in the lower ranges of the DMT Magic Eye level the texture of the Chrysanthemum tends to exhibit heptagonal and triheptagonal tilings (as shown in the picture above). A explains that at the critical point between the Chrysanthemum and the Magic Eye levels the intensity of the rate of symmetry detection of the Chrysanthemum cannot be contained to a 2D surface. Thus, the surface begins to fold, often in semi-symmetric ways. Every time one “recognizes” an object on this “folding Chrysanthemum” the extra curvature is passed on to this object. As the dose increases, one interprets more and more of this extra curvature and ends up shaping a complex and highly dynamic spatiotemporal depth map with hyperbolic folds(“Crochet,” n.d.). In the upper ranges of the Magic Eye level the world-sheet is so curved that the scenes one visualize are intricate and expansive, feeling at times like one is able to peer through one’s horizon in all directions and see oneself and one’s world from a distance. At some critical point one may feel like the space around one is folding into a huge dome where the walls are made of whatever texture + world-sheet combination happened to win the Darwinian selection pressures applied to the qualia patterns on the Magic Eye level. This concentrated hyperbolic synesthetic texture is what becomes the walls of the Waiting Room…
Waiting Room
In the range of 12-25mg of DMT a likely final destination is the so-called Waiting Room. This experience is distinguished from the Magic Eye level in several ways: first, the world-sheet at this level breaks into several quasi-independent components, each evolving semi-autonomously. Second, one goes from “partial immersion” into “full immersion.” The transition between Magic Eye and Waiting Room often looks like “finding a very complex element in the scene and using it as a window into another dimension.” The total 2D surface curvature present (by adding up the curvature of all elements in the scene) is substantially higher than that of the Magic Eye level, and one can start to see actual 3D hyperbolic space. Perhaps a way of describing this transition is as follows: Thecurvature of the world-sheet gets to be so extreme that in order to accommodate it one’s entire multi-modal experiential field becomes involved, and a feeling of total and complete synchronization of all senses into a unified synesthetic experience is inescapable (often described as the “mmmMMMMMMM+++++!!!” whole-body tone people report). Thus the feeling of entering into an entirely new dimension. This explains what people mean when they say: “I experienced such an intense pressure that my soul could not be contained in my tiny body, and the intense pressure launched me into a bigger world.”

The images above, taken together, are meant as an impressionistic replication of what a Waiting Room experience may feel like. On the left you see the textured world-sheet curved in several ways resulting in an enclosed room with shimmering walls and an entity looking at a futuristic-looking contraption. The images on the right are meant to illustrate the ways in which the texture of the world-sheet evolves: you will find that the micro-structure of such texture is constantly unfolding in new symmetrical ways (bottom right), and propagating such changes throughout the entire surface at a striking speed (top right).
DMT Waiting Rooms contain entities that at times do interact directly with you. Their reality is perceived as a much more intense and intimate version of what human interaction normally is, but they do not give the impression of being telepathic. That said, their power is felt as if they could radiate it. One could say that this level of DMT places you in such an intimate, vulnerable and open state that interpreting the entities in a second-person social mode is almost inevitable. It is like interacting with someone you really know (or perhaps someone you really really want to know… or really really don’t want to know), except that the whole world is made of those feelings and some entities inhabit that world.
Serious hard-core psychonauts tend to describe the Waiting Room as a temporary stopgap. Indeed more poetry could ever be written about the Waiting Room states of consciousness than about most human activities, for its state-space is larger, more diverse and more hedonically loaded. But even so, it is important to realize that there are even weirder states. Serious psychonauts exploring the upper ranges of humanly-accessible high energy consciousness research may see Waiting Rooms as a stepping stones to the real deal…
Breakthrough
If one manages to ingest around 20-30mg of DMT there is a decent chance that one will achieve a DMT breakthrough experience (some sources place the dosage as high as 40mg). There is no agreed-upon definition for a “DMT breakthrough,” but most experienced users confirm that there is a qualitative change in the structure and feel of one’s experience on such high doses. Based on A’s observations we postulate that DMT breakthroughs are the result of a world-sheet with a curvature so extreme that topological bifurcations start to happen uncontrollably. In other words, the very topology of one’s world-sheet is forced to change in order to accommodate all of the intense curvature.



The geometry of space you experience may suddenly go from a simply connected space into something else. What does this mean? Suddenly one may feel like space itself is twisting and reconnecting to itself in complex (and often confusing) ways. One may find that given any two points on this “alien world” there may be loops between them. This has drastic effects on one’s every representation (including, of course, the self-other divide). The particular feeling that comes with this may explain the presence of PSIS-like experiences induced by DMT and high dose LSD (cf. LSD and Quantum Measurements). Since the topological bifurcations are happening on a 3D1T world-sheet, this may look like “multiple things happening at once” or “objects taking multiple non-overlapping paths at once in order to get from one place into another.” The entities at this level feel transpersonal: due to the extreme curvature it is hard to distinguish between the information you ascribe to your self-model and the information you ascribe to others. Thus one is all over the place, in a literal topological sense.
While on the Waiting Room one can stabilize the context where the experience seems to be taking place, on a DMT breakthrough state one invariably “moves across vast regions, galaxies, universes, realities, etc.” in a constant uncontrollable way. Why is this? This may be related to whether one can contain the curvature of the objects one attends to. If the curvature is uncontrollable, it will “pass on to the walls” and result in constant “context switches.” In fact, such a large fraction of 3D space is perceived as hyperbolic in one way or another, that one seems to have access to vast regions of reality at the same time. Thus a sense of radical openness is often experienced.
Amnesia Level
Unlike 5-MeO-DMT, “normal DMT” experiences are not typically so mind-warping that they dissolve one’s self-model completely. On the contrary, many people report DMT as having “surprisingly little effect on one’s sense of self except at very high doses” relative to the overall intensity of the alteration. Thus, DMT usually does not produce amnesia due to ego death directly. Rather, the amnesic properties of DMT at high doses can be blamed on the difficulty of instantiating the necessary geometry to make sense of what was experienced. In the case of doses above “breakthrough experiences” there is a chance that the user will not be able to recall anything about the most intense periods of the journey. Unfortunately, we are not likely to learn much from these states (that is, until we live in a community of people who can access other phenomenal geometries in a controlled fashion).
Recalling the Immemorial
We postulate that the diffculty people have remembering the phenomenal quality of a DMT experience is in part the result of not being able to access the geometry required to accurately relive their hallucinations. The few and far apart elements of the experience that people do somehow manage to remember, we posit, are those that happen to be (relatively) easy to embed in 3D Euclidean space. Thus, we predict that what people do manage to “bring back” from hyperspace will be biased towards those things that can be represented in R3.
This explains why people remember experiencing intensely saddled scenes (e.g. fractals, tunnels, kale worlds, recursive processes, and so on). Unfortunately most information-rich and interesting (irreducible, prime) phenomenal objects one experiences on DMT are by their very nature impossible to embed in our normal experiential geometry. This problem reveals an intrinsic limitation that comes from living in a community of intelligences (i.e. contemporary humans) who are constrained in the range of state-spaces of consciousness that they can access. This realization calls for a new epistemological paradigm, one that incorporate state-specific representations into a globally accessible database of states of consciousness(Emilsson 2016b), together with the network that emerges from their mutual (in)intelligibility.
DMT Objects
The increased curvature of one’s world-sheet can manifest in endless ways. In some important ways, the state-space of possible scenes that you can experience on DMT is much bigger than what you can experience on normal states of consciousness. Strictly speaking, you can represent more scenes on DMT states than in most other states because the overall amount qualia available is much larger. Of course the very dynamics of these experiences constrains what can be experienced, so there are still many things inaccessible on DMT. For instance, it may be impossible to experience a perfectly uniform blue screen (since the Chrysanthemum texture is saturated with edges, surfaces and symmetrical patterns). Likewise, scenes that are too irregular may be impossible to stabilize given the omnipresent symmetry enhancement found in the state.
What are the nature of the objects and entities one experiences on DMT? Magic Eye level experiences tend to include objects that are usually found in our everyday life. It is at the DMT waiting room level and above that the “truly impossible objects” begin to emerge. In particular, all of these objects are often curved in extreme ways. They condense within them complex networks of interlocking structures sustaining an overall superlative curvature. Here are some example objects that one can experience on Waiting Room and Breakthrough level experiences:



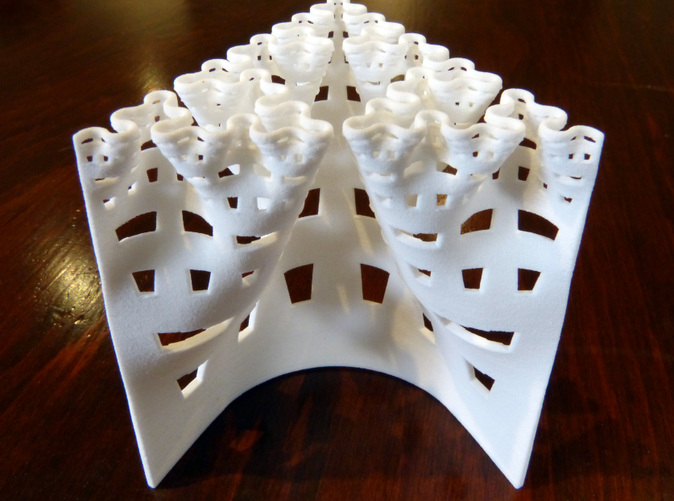


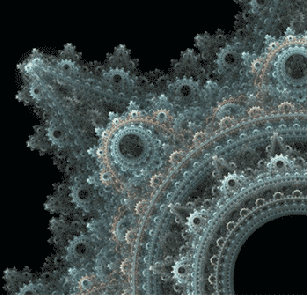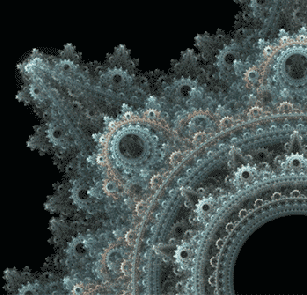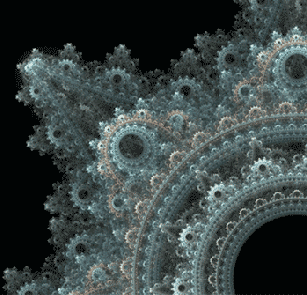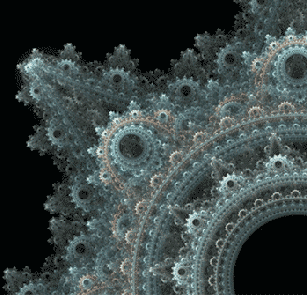


DMT Object 1, 2, 3, 5 - © Paul Nylander, http://bugman123.com
DMT Object 8 - Copyright 2014 Robert Fathauer; robertfathauer.com
Notice that all of these images have many saddles everywhere. Ultimately, the range of objects one can experience on such states includes many other features that are impossible to represent in R3. The objects that people do manage to bring back and recall later on, are precisely those that can be embedded in R3. Thus you often see extremely contorted wrapped-up objects. The most interesting ones (such as quasi-regular H3 tilings or irreducible objects) are next-to-impossible to bring back in any meaningful way, for now at least.
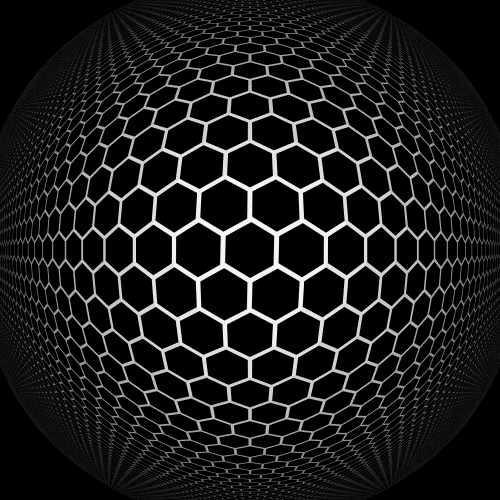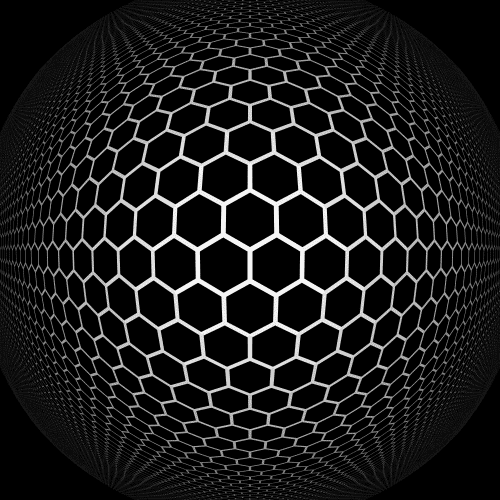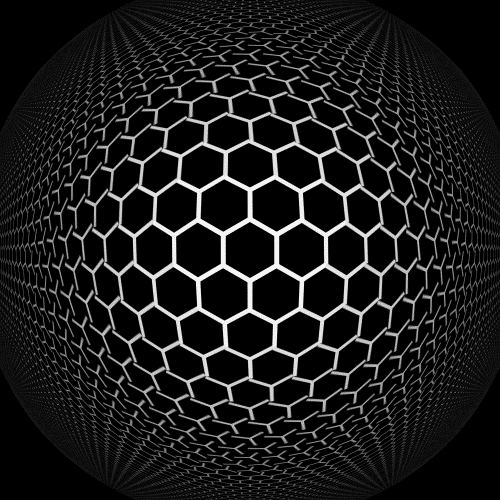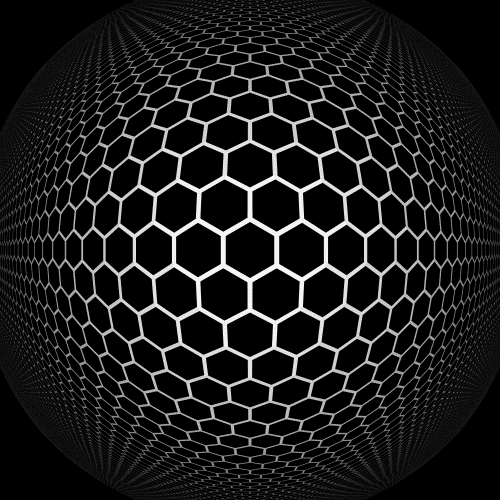
DMT Space Expansion
The expansion of space responsible for the increased curvature happens anywhere you direct your attention (including the objects you see). Here(“Jitterbox,” n.d.) you can see what it may look like to stare at a DMT object. This is called the “jitterbox” mechanism.
DMT Entities
DMT entities come in many forms, and their overall quality is extremely dosedependent. Rather than describing any specific manifestation we will instead briefly characterize the rough properties of the entities experienced based on the level reached.
- Threshold: Usually the ambiance change has a social feel to is. More similar to entering a room of people of an alien culture, than entering an empty cave or a warm pool on your own. In this sense the very beginning of a DMT experience may already frame the experience in social terms and facilitate the expectation of meeting entities.
- Chrysanthemum: One can feel perhaps the subtle presence of entities, but they are often interpreted as “feeling connected” to one’s friends, relatives and acquaintances. The feeling does not manifest in any clear spatial way, though. Other than that, this state is apersonal in the sense that one does not see any entity directly.
- Magic Eye: Here the entities can be roughly described as having an impersonal relationship with you. They are just there, hanging out on their own, often engrossed with whatever activities your world-sheet is capable of representing for them.
- Waiting Room: At this level entities start becoming able to interact with you. They feel like autonomous beings wrapped in mystery. Their intentions, what they know, and their emotional states can be guessed from their behavior, but they are not immediately obvious.
- Breakthrough: At this level the entities one meets seem to have what we might call a transpersonal relationship with you. They share their own internal states (emotions, knowledge, wishes, etc) with you. It feels like they can communicate telepathically and “see through” you. One cannot hide one’s “private” mental contents from them at this level.
- Amnesia: One cannot remember, of course, exactly what happens here. But if trip reports are any indication, this level is reminiscent of highly “mystical” states in which one’s implicit beliefs about Personal Identity(Emilsson 2015e) are obliterated and replaced by the feeling of becoming an all-encompassing entity. “Union with God” and “Samadhi(Emilsson 2016d)” are terms that describe the subjective feeling of self in this state. In other words, at this level it is impossible to distinguish between oneself and other entities, for all is represented as one. (Beware of never trying to go here if you feel bad at the time since negative hedonic tone can be amplified just as much as a good feeling such as Samadhi).
Modeling the Hyperbolic Geometry of DMT
How can we explain the drastic geometric changes of phenomenal space on DMT? As mentioned earlier, we will discuss three (non-mutually exclusive) hypothesis. These hypothesis work at the level of an algorithmic reduction(Emilsson 2016a), which means that we will go deeper than just describing information processing and phenomenology. We will stop short of addressing the implementation level of abstraction. It is worth pointing out that describing the ways in which DMT experiences are hyperbolic is in itself an algorithmic reduction. What we are about to do is to develop a more granular algorithmic reduction in which we try to explain why hyperbolic geometry emerges on DMT states by postulating underlying processes. Here are the three reductions:
Control Interruption + Symmetry detection = Change in Metric
Recall that on a previous article(Emilsson 2016a) we algorithmically reduced general psychedelic states. The building blocks of that reduction were:
- Control Interruption(“The Control Interrupt Model of Psychedelic Action,” n.d.) (which amounts to a “longer half-life for all qualia”)
- Drifting (“breathing walls, eyes moving from their normal place, waving sensations”)
- Enhanced Pattern Recognition (pareidolia, cf. Getting Closer to Digital LSD(Emilsson 2015d))
- Lowered Symmetry Detection Threshold (quasi-symmetric patterns tend to “lock into” perfectly symmetrical structures)
Using this framework one can argue that DMT makes space more hyperbolic in the following way: in high amounts the synergistic effect of control interruption(“The Control Interrupt Model of Psychedelic Action,” n.d.) together with extremely lowered symmetry detection thresholds experienced in quick succession makes the subjective distance between the points in the phenomenal objects in the scene evolve a hyperbolic metric. How would this happen? The key thing to realize is that in this model the usual quasi-Euclidean space we experience is an emergent effect of an equilibrium between these two forces. Even in normal circumstances our world-sheet is continuously regenerated; the rate at which symmetrical relationships in the scene are detected is balanced by the rate at which these subjective measurements are forgotten. This usually results in an emergent Euclidean geometry. On DMT the rate of symmetry detection increases while the rate of “forgetting” (inhibiting control) decreases. Attention points out more relationships in quick succession and this creates a network of measured subjective distances that cannot be embedded in Euclidean 3D space. Thus there is an overflow of symmetries. We are currently working on a precise mathematical model of this process in order to reconstruct a hyperbolic metric out of these two parameters. In this model, control interruption is interpreted as a change in the decay for subjective measurements of distance in one’s mind, whereas the lowered symmetry detection threshold is interpreted as a change in the probability of measuring the distance between any two given points as a function of the network of distances already measured.
The curvature increase is most salient where there is already a lot of measurements made, since highly-measured regions focus attention and attention drives symmetry detection. Thus, focusing on any surface will make the surface itself hyperbolic (rather than the 3D space, since measurements are mostly concentrated on the surface). On the other hand, if the curvature is too high to keep on a 2D surface, it will “jump” to 3D or even 3D1T (i.e. branching out the temporal component of one’s experience). The result is that the total curvature of one’s 3D1T world-sheet increases on DMT in a dose-dependent way.
Different doses lead to different states of curvature homeostasis. Each part of the worldsheet has constantly-morphing shapes and sudden curvature changes, but the total curvature is nonetheless more or less preserved on a given dose. It is not easy to get rid of excess curvature. Rather, whenever one tries to reduce the curvature in one part of the scene one is simply pushing it elsewhere. Even when one manages to push most of the curvature out of a given modality (e.g. vision) it is likely to quickly return in another modality (e.g. kinesthetic or auditory landscape) since attention never ceases on a DMT trip. Such apparent dose-dependent global curving of the world-sheet (and its jump from one modality into another) constrains the shape of the objects one can represent on the state (thus leading to alien-looking highly-curved objects similar to the ones shown above).
Dynamic System Account: Energy Sources, Sinks and Invariants
Energy Invariants
Let us define a notion of energy in consciousness so that we can formalize the way experiences warps and transforms on DMT. Assume that one needs “energy” in order to instantiate a given experience (really, this is just an implicit invariant and we could use a different name). Each feature of a given experience needs a certain amount of energy, which roughly corresponds to a weighted sum of the intensity and the information content of an experience. For instance, the brightness of a point of colored light in one’s visual field is energy-dependent. Likewise, the information content in a texture, the number of represented symmetrical relationships, the speed by which an object moves (plus its acceleration), and even the curvature of one’s geometry. All of these features require energy to be instantiated.
Under normal circumstances the brain has many clever and (evolutionarily) appropriate ways of modulating the amount of energy present in different modules of one’s mind. That is, we have many programs that work as energy switches for different mental activities depending on the context. When we think, we have allocated a certain amount of energy to finding a shape/thought- form that satisfies a number of constraints. When it shape-shifting that energy in various ways and finding a solution, we either allocate more energy to it or perhaps give up. However, on DMT the energy cannot be switched off, and it can only pass from one modality into another. In other words, whereas in normal circumstances one uses strategically one’s ability to give energy limits to different tasks, on DMT one simply has constant high energy globally no matter what.
More formally, this model of DMT action says that DMT modifies the structure of one’s mind so that (1) energy freely passes from one form into another, and (2) energy floods the entire system. Let’s talk about energy sources and sinks.
Energy Sources and Sinks
In this algorithmic reduction DMT increases the amount of consciousness in one’s mind by virtue of impairing our normal energy sinks while increasing the throughput of its energy sources. This may frequently manifests as phenomenal spaces becoming hyperbolic in the mathematical-geometric sense of increasing its negative curvature as such curvature is one manifestation of higher levels of energy. Energy sinks are still present and they struggle to capture as much of the energy as possible. In particular, one energy sink is “recognition” of objects on the world-sheet. This model postulates that attention functions as an energy source, whereas pattern recognition functions as an energy sink.
The Hamiltonian of a World-Sheet
The total energy in one’s consciousness increases on DMT, and there is a constant flow between different ways for this energy to take form. That said, one can analyze piecewise the various components of one’s experience, specially if the network of energy exchange clusters well. In particular, we can postulate that world-sheets are fairly self-contained. Relative to other parts of the environment the mind is simulating, the world-sheet itself has a very high within-cluster energy exchange and a relatively low cross-cluster energy exchange. One’s world-sheet is very fluid, and little deformations propagate almost linearly throughout it. In a given dose plateau, if you add up the acceleration, the velocity, the curvature, and so on of every point in the world-sheet you will come up with a number that remains fairly constant over time. Thus studying the Hamiltonian of a world-sheet (i.e. the state-space given by a constant level of energy) can be very informative in describing both the information content and the experiential intensity of DMT experiences.

Under normal circumstances the curvature of one’s world-sheet is, as far as I can tell, arousal-dependent. Have you noticed how when you feel tired you are more likely to defocus your visual experience? You are tired late at night and you are trying to watch a movie, but bringing the scene in focus is too much of an effort so you defocus for a little bit (still listening to the dialogue). What did you do that for? In the framework here proposed, you did that to diminish the energy it takes you to sustain a curved world-sheet with a lot of information. Doing so may be aesthetically pleasing and rewarding when fully awake or excited, but when tired the returns on doing the focusing are not great given how much effort it needs and the fact that the dialogue is more essential for the plot anyway.
It takes effort and wakefulness to focus on a complex scene with many intricate details. (Reading and trying to comprehend this essay may itself requires ignificant conscious energy expenditure). For this reason we might say that DMT is an exceedingly effective arouser of consciousness.
Bayesian Energy Sinks
One essential property of our minds is that our level of mental arousal decreases when we interpret our experience as “expected.” People who can enjoy their own minds do so, in part, by finding unexpected ways of understanding expected things. In the presence of new information that one cannot easily integrate, however, one’s level of energy is adjusted upwards so that we try out a variety of different models quickly and try to sort out a model that does make the new information expected (though perhaps integrating new assumptions or adding content in other ways). When we cannot manage to generate a mental model that works out a likely model of what we are experiencing we tend to remain in an over-active state.
This general principle applies to the world-sheet. One of the predominant ways in which a world-sheet reduces its energy (locally) is by morphing into something you can recognize or interpret. Thus the world-sheet in some way keeps on producing objects, at first familiar, but in higher energies the whole process can seem desperate or hopeless: one can only recognize things with a stretch of the imagination. Since humans in general lack much experience with hyperbolic geometry, we usually don’t manage to imagine objects that are symmetric on their own native geometry. But when we do, and we fill them up with resonant light-mind-energy, then BAM! New harmonics of consciousness! New varieties of bliss! Music of the angels! OMG! Laughter till infinity and more- shared across the galaxy- in a hyperbolic transpersonal delight! It’s like LSD and N20(“On LSD and Nitrous Oxide - the Psychedelic Experience - Shroomery Message Board,” n.d.)! Wow!
Forgive me; it is my first day. Let’s carry on. As one does not know any object that the world-sheet can reasonably be able to generate in high doses, and the world-sheet has so much energy on its own, energy can seem to spiral out of control. This explains in part the non-linear relationship between experienced intensity and DMT dose.
Like all aspects of one’s consciousness, the negative curvature of phenomenal space tends to decay over time (possibly through inhibition by the cortex). In this case, the feeling is one of “smoothing out the curves” and embedding the phenomenal objects in 3D euclidean space. However, this is opposed by the effect that attention and (degrees of ) awareness have on our phenomenal sheet, which is to increase its negative curvature. On DMT, anything that attention focuses on will begin branching, copying itself and multiplying, a process that quickly saturates the scene to the point of filling more spatial relationships than would fit in Euclidean 3D. The rate at which this happens is dose-dependent. The higher the dose, the less inhibiting control there is and the more intense the “folding” property of attention will be. Thus, for different dosages one reaches different homeostatic levels of overall curvature in one’s phenomenal space. Since attention does not stop at any point during a DMT trip (it keeps being bright and intense all throughout) there isn’t really any rest period to sit back and see the curvature get smoothed out on its own. Everything one thinks about, perceives or imagines branches out and bifurcate at a high speed.
Every moment during the experience is very hard to “grasp” because the way one normally does that in usual circumstances is by focusing attention on it and shaping one’s world-sheet to account for the input. But here that very attention makes the world-sheet wobble, warp and expand beyond recognition. Thus one might say that during a solid DMT experience one never sees the same thing twice, as the experience continues to evolve. That is, of course, as long as you do not stumble upon (or deliberately create) stable phenomenal objects whose structure can survive the warping effect of attention.
Hyperbolic Micro-Structure of Consciousness
Subjectively, A says, negative curvature is associated with more energy. Perhaps this curvature happens at a very low level? An example to light up the imagination is using heat to fold a sheet of metal(“Thermal Expansion and Deformation of Aluminum Sheet,” n.d.) (thanks to thermal expansion). Whatever your attention focuses on seems to get heated up (in some sense) and expand as a result. The folding patterns themselves seem to store potential energy. Left on their own, this extra energy stored as negative curvature usually dissipates, but on DMT this process is lowered (while the effect of increasing the energy is heightened). Could this be the result of some very very fine-level micro-experiential change that gradually propagates upwards? With the help of our normal mental processes the change in the micro-structure may propagate all the way into seemingly hyperbolic 2D and 3D surfaces.
Perhaps the most important difference between DMT in high doses and other psychedelics is that the micro-structure of consciousness drifts in such a way that tiny Droste effects bubble up into large M¨obius transforms(“Spherical Video Editing with Möbius Transformations,” n.d.).
As noted already, these three algorithmic reductions are not incompatible. We just present them here due to their apparent explanatory power. A lot more theoretical work will be needed to make them quantitative and precise, but we are optimistic. The aim is now to develop an experimental framework to distinguish between the predictions that each candidate algorithmic reduction makes (including many not presented here). This is a work in progress.
Generalizing hyperbolization to non-spatial experiential fields
In the case of experiential fields such as body feelings, smells and concepts, the “hyperbolization” takes different forms depending on the algorithmic reduction you use. I prefer the very general interpretation that one experiences hyperbolic information geometry rather than just hyperbolic space. In other words, when we talk about body feelings and so on, on a psychedelic one organizes such information in a hyperbolic relational graph, which also exhibits a negative curvature relative to its normal geometry. Arguing in favor of this interpretation would take another article, so we will leave that for another time.
Getting a handle on the DMT state
Gluing a 1-handle is easy on a 2-sphere. Tongue in cheek, sticking a little doughnut on a big ball allows you to grab the sphere and control it in some way. But how do you get a handle on hyperbolic space? The answer is to build hyperbolic manifolds at the core of one’s being, by imagining knots very intensely. The higher one is, the more complex the knot one can imagine in detail. Having practiced visualizations of this sort while sober certainly helps. If you imagine the knot with enough detail, you can then stress the environment surrounding it to represent a warped hyperbolic space. This way you give life to the complement of the knot(“Not Knot,” n.d.) (which is almost always hyperbolic!). We postulate that it is possible to study in detail the relationship between the knots imagined, and the properties of the experiential worlds that result from their inversion (i.e. thinking about the geometry of the space surrounding the knot rather than the knot itself ). A reports that different hyperbolic spaces generated this way (i.e. imagining knots on tryptamines) have different levels of energy, and have unique resonant properties. Different kinds of music feel better in different kinds of hyperbolic manifolds. It takes more energy to “light up” a hyperbolic space like that, mostly due to its openness. This is why using small doses of 2C-B can be helpful to create a positive backbone to the experience (providing the necessary warmth to light up the hyperbolic space). Admittedly MDMA tends to work best, but its use is unadvisable for reasons we will not get into (related to the hedonic treadmill). A healthy combination that both enables the visualization of the hyperbolic spaces in a vivid way and also lights them up with positive hedonic tone healthily and reliably has yet to be found.
Relatedly… Get a handle on your DMT trip by creating a stabilizing 4D hyperbolic manifold in four easy steps:
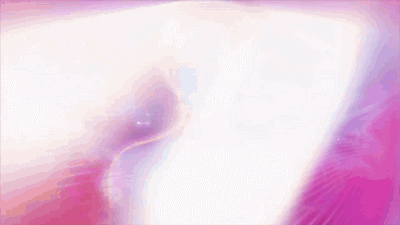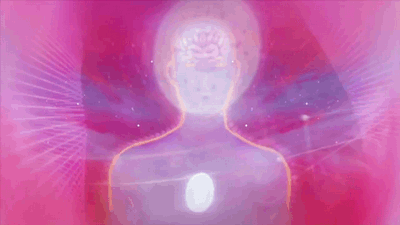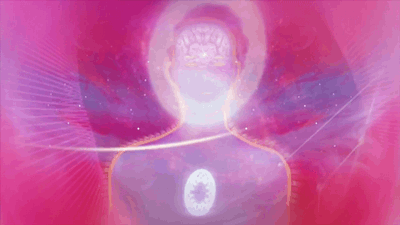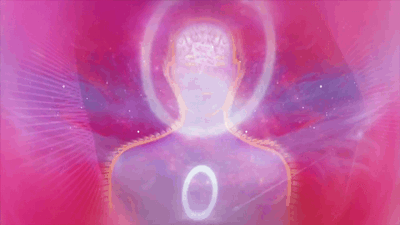



Unifying Your Space
God, the divine, open individualism, the number one, an abstract notion of self, or the thought of existence itself are all thoughts that work as great “unifiers” of large areas of phenomenal space. Indeed these concepts can allow a person to connect the edges of the hyperbolic space and create a pocket of one’s experience that does not seem to have a boundary yet is extremely open. This may be a reason why such ideas are very common in high levels of psychedelia. In a sense, depending on the mind, they have at times the highest recruiting power for your multi-threaded attention.
Applications to Qualia Computing and Closing Thoughts
Beyond mere designer synesthesia, the future of consciousness research contains the possibility of exploring alternative geometries for the layout of our experiences. One’s overall level of energy, its manifestation, the allowed invariants, the logic gates, the differences in resonance, the granularity of the patterns, and so on, are all parameters that we will get to change in our minds to see what happens (in controlled and healthy ways, of course). The exploration of the state-space of consciousness is sure to lead to a combinatorial explosion. Even with good post-theoretical quantitative algorithmic reductions, it is likely that qualia computing scientists will still find an unfathomable number of distinct “prime” permutations. For some applications it may be more useful to use special kinds of hyperbolic spaces (like the compliment of certain class of knot), but for others it may suffice to be a little sphere. Who knows. In the end, if a valence economy ends up dominating the world, then the value of hyperbolic phenomenal spaces will be proportional to the level of well-being and bliss that can be felt in them. Which space in which resonant mode generates the highest level of bliss? This is an empirical question with far-reaching economic implications.
Mathematics post-hyperbolic consciousness
I predict that some time in the next century or so many of the breakthroughs in mathematics will take place in consciousness research centers. The ability to utilize arbitrary combinations of qualia with programable geometry and information content (in addition to our whole range of pre-existing cognitive skills) will allow people to have new semantic primitives related to mathematical structures and qualia systems currently unfathomable to us. In the end, studying the mathematics of consciousness and valence is perhaps the ultimate effective altruist endeavor in a world filled with suffering, since reverse-engineering valence would simplify paradise engineering… But even in a post-scarcity world, consciousness research will also probably be the ultimate past time given the endless new discoveries awaiting to be found in the state-space of consciousness.
P.S. Note on Originality: The only mention I have been able to find that explicitly connects hyperbolic geometry in a literal sense with DMT (rather than just metaphorical talk of “hyperspace”) is a 2014 post in the Psychonaut subreddit(“R/Psychonaut - DMT, Hyperbolic Space, and Dodecahedrons,” n.d.). To my knowledge, no one has yet elaborated to any substantial degree on this interesting connection. That said, I’m convinced that during the days that follow a strong trip, psychedelic self-experimenters may frequently wonder about the geometry of the places they explored. Yet they usually lack any conceptual framework to justify their intuitions or even verbalize them, so they quickly forget about them.
P.S.S. Example Self-Dribbling Basketball: Below you can see what a “self-dribbling basketball” looks like. The more you try to “grasp” what it is, the more curved it gets. That’s because you are adding energy with your attention and you do not have enough recognition ability in this space to lower its energy and reduce the curvature to stabilize it. The curvature is so extreme at times that it produces constant “context switches.” This is the result of excess curvature being pushed towards the edge of your experience and turning into walls and corridors.
Acknowledgements
Thank you James W. Sanders for converting this piece to Distill for R Markdown format.
Tags
Psychedelics, Philosophy, Qualia, Ethics, Experiment, Hedonic Tone, Psychophysics, Symmetry, Valence
On the unexpected side effects of staring at a cauliflower on DMT: You can get lost in the hyperbolic reality of the (apparent) life force that spirals in a scale-free fractal fashion throughout the plant. The spirals may feel like magnetic vortexes that take advantage of your state to attract your attention. The cauliflower may pull you into its own world of interconnected fractals, and as soon as you start to trust it, it begins trying to recruit you for the cauliflower cause. The cauliflower may scare you into not eating it, and make you feel guilty about frying it. You may freak out a little, but when you come down you convince yourself that it was all just a hallucination. That said, you secretly worry it was for real. You may never choose to abstain from eating cauliflowers, but you will probably drop the knife when cooking it. You will break it apart with your own hands in the way you think minimizes its pain. You sometimes wonder whether it experiences agony as it is slowly cooked in the pan, and you drink alcohol to forget. Damn, don’t stare at a cauliflower while high on DMT if you ever intend to eat one again.↩︎
